Tunnel – FHWA InfoTechnology

Tunnel - Vibration Testing (VT)

Target of Investigation

Vibration testing can be used to analyze the behavior of wires, cables, hangers, truss members, and other slender structural members of tunnels. Vibration testing has the following applications:

Estimate the force carried by the member under inspection; this identifies members that may have damaged connections or section loss from corrosion.⁽¹⁾
Assess load distributions in a structure and identify irregularities resulting from unexpected settlements or construction errors.⁽²⁾
Evaluate unanticipated vibrations caused by weather-related phenomena, such as
wind–rain interactions or wind-induced resonant vibrations.^(3,4)

Description

Vibration testing typically consists of measuring the vibration of the member under inspection using an accelerometer, laser vibrometer, or digital image–processing technique. Time records of member vibrations are analyzed to determine the magnitude and frequency characteristics of the vibrations. The vibration frequency can be compared to mathematical models to estimate the force carried in the member. Typically, the force is estimated to determine if a given member is carrying an increased or decreased load as a result of damage. For example, in a tunnel with ceiling panels, the load levels carried in different ceiling-panel hangers can be used to identify hangers that are not carrying the anticipated loading owing to damage to the hanger or its anchorages. The amplitude and frequency characteristics of vibrations can also be used to analyze high-amplitude vibrations that occur as a result of member resonance.

Physical Principle

The physical principle of vibration testing to analyze force in a member is based on the relationship between the force carried in a member and its resonant frequency of vibration. A taut-string analogy is often used to describe this phenomenon. The equation in figure 1 shows vibration frequency as a function of length, mass, and tension force. If the length and mass of the member are known constants, the tension can be estimated by measuring the resonant frequency.

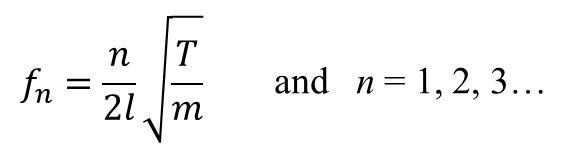
f subscript n equals the product of n divided by 2l times the square root of T divided by m, with n defined as any integer greater than zero. — Figure 1. Equation. Resonant frequency of a taut string.

Where:

f_n = resonant frequency, with n = 1,2,3…

l = length.

T = tension force.

m = mass per unit length.

This equation demonstrates the basic principle for estimating the force carried in a member for a very simple case and does not account for the stiffness or geometry of the member. Analyses that consider factors such as member stiffness and geometric effects (e.g., cable sagging or inclination) can be found in the literature.⁽⁵^–⁸⁾ Vibration testing for the purpose of evaluating weather-related phenomena is based on the principle that certain weather conditions can excite modes of resonant vibration in the high-amplitude range.

Data Acquisition

Data acquisition typically consists of collecting time records of the vibration of the member under inspection. These data can be acquired using accelerometers, a laser vibrometer, or digital image–processing techniques.⁽⁹^–¹¹⁾ Accelerometers are commonly used if continuous monitoring of vibrations is desired. The use of an accelerometer requires the installation of sensors on the member under inspection and a suitable data acquisition system to collect data. Figure 2 shows an accelerometer in use on a concrete prism specimen to collect resonance-frequency data under an impact load. Laser vibrometers and digital-image processing are commonly used for periodic field measurements or for a single test.⁽¹⁾ Both laser vibrometers and digital-image processing are noncontact technologies that do not require the installation of sensors on the member under inspection.

This photo is of a concrete prism on a support. An engineer is about to tap the prism’s top surface close to the right end with an impact hammer. An accelerometer rests on the prism’s top surface. — Source: FHWA.
Figure 2. Photo. Resonance-frequency testing of a concrete prism.⁽¹²⁾

Data Processing

Data are processed to determine the amplitude of vibrations and to identify resonant frequencies of the member under inspection. Fast Fourier transforms (FFTs) are typically used to transform time-domain data (figure 3) to frequency-domain data (figure 4) so that resonant frequencies can be identified.

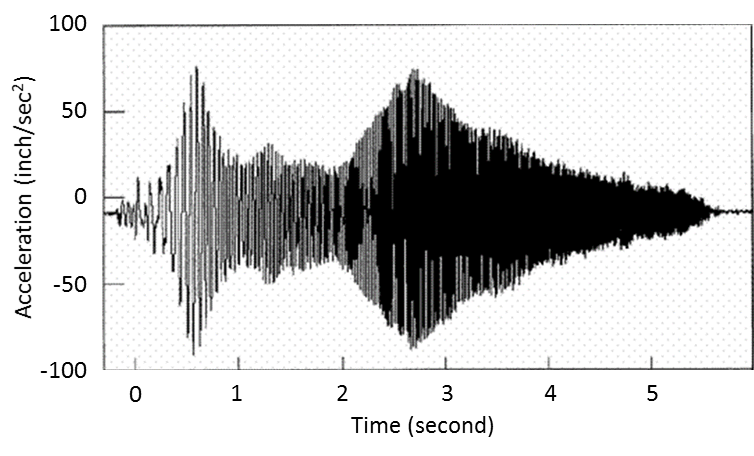
This graph depicts time-domain data collected from vibration testing. The x-axis is time ranging from 0 to 6 seconds. The y-axis is acceleration ranging from negative 100 to positive 100 inches per square second. The data are of very high frequency. Two major peak amplitudes are at 0.5 and 2.7 seconds. — Source: FHWA.
1 inch/s² = 0.0254 m/s².
Figure 3. Graph. Time-domain data from vibration testing.⁽¹³⁾

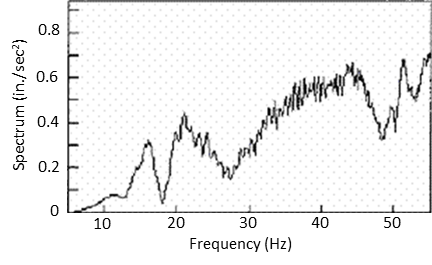
This graph depicts frequency-domain data acquired from a fast Fourier transform of time-domain data. The x-axis is frequency, ranging from 5 to 55 hertz. The y-axis is spectrum, ranging from 0 to 0.95 inches per square second. The data plot increases as the frequency increases; there are peaks at 16, 21, 45, and 51 hertz. — Source: FHWA.
1 inch/s² = 0.0254 m/s².
Figure 4. Graph. Frequency-domain data from FFTs.⁽¹³⁾

Data Interpretation

The interpretation of data from vibration measurements may be qualitative or quantitative. For qualitative analysis, the vibration characteristics of several similar members are compared to determine the relative loading carried by each member. This interpretation can identify members that are carrying unexpected loads and require further analysis or inspection. Figure 5 shows the flexibility spectrums from vibration testing of three piles under different conditions (intact, excavated, and broken). The flexibility spectrum of the intact pile differs significantly from the spectrums of the excavated and broken piles.

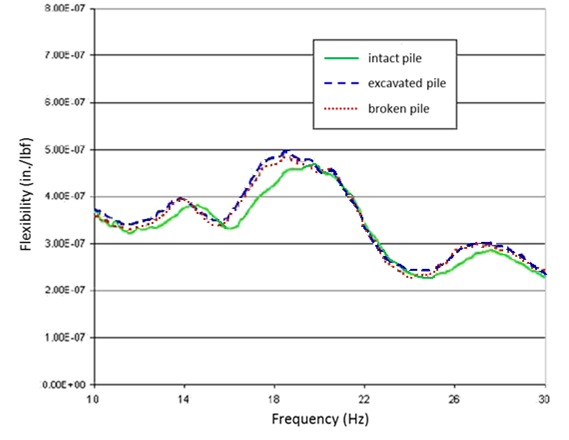
This graph depicts the flexibility spectrums of three piles. The x-axis is frequency ranging from 10 to 30 hertz. The y-axis is flexibility ranging from 0 to 8 all to the power of negative 7 inches per pound force. There are data plots representing the spectrums of an intact pile, an excavated pile, and a broken pile. Each of the three plots has peaks and troughs that are generally similar but not identical. The highest peak for all three plots is at approximately 18 hertz. — Source: FHWA.
1 inch/lbf = 0.0057 m/N.
Figure 5. Graph. Flexibility spectrums of three piles from vibration testing.⁽¹³⁾

Quantitative analysis can be used to estimate the actual forces carried by the member under inspection. Vibration data are compared to a model based on design loadings, allowing variations to be identified. This approach can be used to characterize global behavior, identify members that may be damaged, or analyze unexpected load distributions that may have resulted from support settlement or construction errors.

Data from vibration testing may also be used to characterize the vibration behavior of members that have been exposed to certain weather conditions, such as high winds or wind–rain interactions. These data may be analyzed to identify weather conditions that result in large-amplitude vibrations. These data may aid in designing suitable damping schemes to mitigate high-amplitude vibrations or assessing the effectiveness of a retrofit intended to mitigate high-amplitude vibrations.

Advantages

Advantages of vibration testing include the following:

Noncontact measurements (using digital-image processing or a laser vibrometer).
Rapid testing.
Quantitative analysis of forces carried by members.

Limitations

A limitation of vibration testing is the following:

Complex analysis is required to produce quantitative force measurements.

References

Tabatabai, H., Mehrabi, A.B., and Wen-huei, P.Y. (1998). “Bridge stay cable condition assessment using vibration measurement techniques.” Proceedings SPIE 3400, Structural Materials Technology III: An NDT Conference, International Society for Optics and Photonics, Bellingham, WA.
Ciolko, A.T. and Yen, P.W. (1999). “An Immediate Payoff from FHWA’s NDE Initiative.” Public Roads, 62(6), Federal Highway Administration, Washington, DC.
Zuo, D., Jones, N.P., and Main, J.A. (2008). “Field observation of vortex- and rain-wind-induced stay-cable vibrations in a three-dimensional environment.” Journal of Wind Engineering and Industrial Aerodynamics, 96(6–7), pp. 1124–1133, Elsevier, Amsterdam, Netherlands.
Washer, G., Ruiz-Fabian, P., and Dawson, J. (2016). Monitoring Vibrations on the Jefferson City Bridge, Missouri Department of Transportation, Jefferson City, MO.
Zui, H., Shinke, T., and Namita, Y. (1996). “Practical Formulas for Estimation of Cable Tension by Vibration Method.” Journal of Structural Engineering, 122(6), pp. 651–656, American Society of Civil Engineers, Reston, VA.
Mehrabi, A.B. and Tabatabai, H. (1998). “Unified Finite Difference Formulation for Free Vibration of Cables.” Journal of Structural Engineering, 124(11), pp. 1313–1322, American Society of Civil Engineers, Reston, VA.
Ren, W.X., Chen, G., and Hu, W.H. (2005). “Empirical formulas to estimate cable tension by cable fundamental frequency.” Structural Engineering and Mechanics, 20(3), pp. 363–380, Techno Press, Daejeon, Korea.
Ricciardi, G. and Saitta, F. (2008). “A continuous vibration analysis model for cables with sag and bending stiffness.” Engineering Structures, 30(5), pp. 1459–1472, Elsevier, Amsterdam, Netherlands.
Kim, S.W. and Kim, N.S. (2013). “Dynamic characteristics of suspension bridge hanger cables using digital image processing.” NDT & E International, 59, pp. 25–33, Elsevier, Amsterdam, Netherlands.
Ji, Y. and Chang, C. (2008). “Nontarget Image-Based Technique for Small Cable Vibration Measurement.” Journal of Bridge Engineering, 13(1), pp. 34–42, American Society of Civil Engineers, Reston, VA.
Nassif, H.H., Gindy, M., and Davis, J. (2005). “Comparison of laser Doppler vibrometer with contact sensors for monitoring bridge deflection and vibration.” NDT & E International, 38(3), pp. 213–218, Elsevier, Amsterdam, Netherlands.
Graybeal, B.A. (2006). Material Property Characterization of Ultra-High Performance Concrete, Report No. FHWA-HRT-06-103, Federal Highway Administration, Washington, DC.
Olson, L.D. (2005). Dynamic Bridge Substructure Evaluation and Monitoring, Report No. FHWA-RD-03-089, Federal Highway Administration, Washington, DC.